The postgraduate students and staff of the Department of Statistics attended Dr Bruno Ebner's highly informative seminar on analyzing point patterns by a threshold transformation to binary images at Nelson Mandela University on Friday, 5 April 2019. Following the seminar the staff and students of Nelson Mandela and Rhodes Universities had interesting discussions about the paper. The Rhodes University postgraduate students then travelled to Bay West Mall where they had ice-skating lessons (with various degrees of success!). The departments would like to thank the South African Statistical Association for their support in hosting this event.
Abstract:
Analyzing point patterns by a threshold transformation to binary images
Bruno Ebner, Institute of Stochastics, Karlsruhe Institute of Technology (KIT), Germany
Serious analysis of spatial point patterns always start with a goodness-of-fit test to detect departure from the
absence of structure in the data. Whether one has a known or a random number of points two different models
apply, namely the uniform distribution (for goodness-of-fit of uniformity, see [3]) and the concept of complete
spatial randomness, which is synonymous for a homogeneous Poisson point process. We propose for the latter
case some new goodness-of-fit procedures for data in a given observation window. Therefor we use an equidistant
binning of the observation window and apply a threshold procedure to generate binary images which are then
analyzed by Minkowski functionals like the area, the perimeter and the Euler characteristic. Examples of the
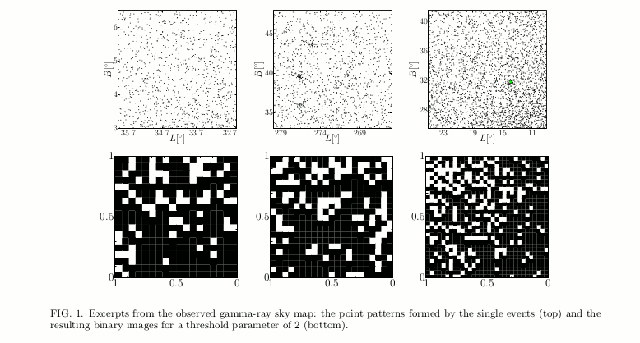
transition to a binary image can be found in Figure 1. We demonstrate that the computation of Minkowski
functionals of binary images is efficient by utilizing techniques of image analysis resulting in a look-up table.
Asymptotic distributional theory is derived under the hypothesis and a suitable limiting regime using a limit
theorem for dependency graphs, incorporating the local dependency structure and representations as sums of
random variables of the Minkowski functionals. Under some specific fixed alternative inhomogeneous Poisson
point processes, we show almost sure limits leading to first consistency statements. We compare the new
methods to existing procedures (as presented in [1]) in a Monte Carlo simulation study. Finally we apply the
new tests to a real data set, namely the gamma-ray sky map of the Fermi Gamma-ray Space Telescope, where
we analyze a sky-map of gamma-rays observed by the so-called Large Area Telescope, see Figure 1 for pictures
of the investigated data sets.
This is joint work with Norbert Henze, KIT, Michael A. Klatt, KIT, and Klaus Mecke, University of Erlangen-
Nurnberg, Germany, see [2].
Corresponding author: bruno.ebner@kit.edu
[1] A. Baddeley, E. Rubak, and R. Turner, Spatial Point Patterns - Methodology and Applications with R (CRC Press,
Boca Raton, Florida, USA, 2016).
[2] B. Ebner, N. Henze, M.A. Klatt, and K. Mecke (2018) ”Goodness-of-fit tests for complete spatial randomness based
on Minkowski functionals of binary images”, Electronic Journal of Statistics, Vol. 12, No. 2, 2873-2904.
[3] B. Ebner, N. Henze, and J.E. Yukich (2018) ”Multivariate goodness-of-fit on flat and curved spaces via nearest
neighbor distances”, Journal of Multivariate Analysis, Volume 165, p. 231-242.
